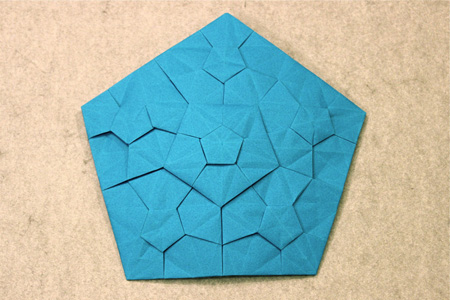
The third in a series of Penrose tessellations, with seventy cells, made by subdividing the layout of the second tessellation. Folded from a pentagonal sheet.

The second in a series of Penrose tessellations, with thirty-five cells, made by subdividing the layout of the first tessellation. Folded from a pentagonal sheet.

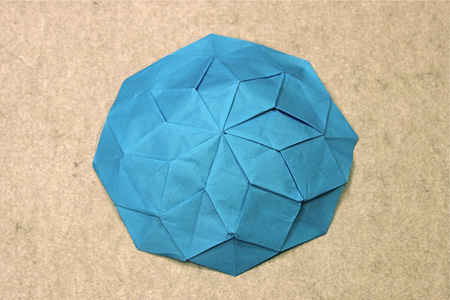
The first in a series of Penrose tessellations, with ten cells. Folded from a pentagonal sheet.

I created this mandala-like pattern at 2011 convention. It's a spiral matrix of parallelograms that features fivefold symmetry. At first I thought it was a Penrose tessellation, but the joining rules do not conform to the Penrose tiling.

A precursor to the Pseudo-Penrose Star (a.k.a. Penflower Mandala). It features a fourfold symmetry that is easer to fold and has fewer cells (24 vs. 40). In fourfold geometry this is an allowable quasicrystal tiling.

A variation on the Pent-Hex tessellation, using only pentagons. The one shown is a second-order fractal, but it could recurse to any depth if the folder has enough patience. Also, this models is flat, but the same pattern could be used to tile a curved surface. A spherical surface would result in a closed polygon with 72 pentagonal bases. The base would be a dodecahedron. This is on my list of things to fold.

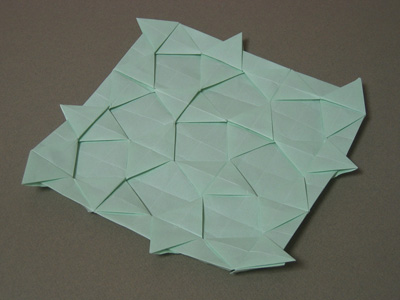
An elaboration on the Cairo Tessellation, this pattern features a grid of alternating pentagons and hexagons.

Tessellations have become very popular in origami. My first tessellation features all pentagons. It doesn't use pentagonal symmetry however, the underlying grid is square. The Cairo tessellation is an ancient pattern used in Moorish and Mideastern art and architecture for centuries.

The grid of triangles and squares is a complement to the Cairo Tessellation. This mesh is the dual of the other. If you draw a dot in the center of each cell of the pentagon of the Cairo grid and connect them all, you get this pattern. Five cells converge at each vertex. In the other pattern all the cells were the same shape, but either three or four cells converged at a vertex. The two of them make a nice set.