Part 2: Fun With Shapes
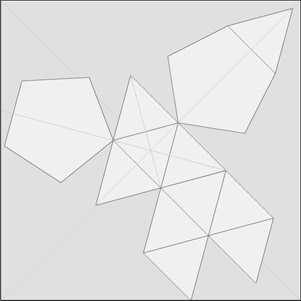
Now the Origami part of the trip: At the Strong Museum there was a place to play with shapes, and I discovered something interesting. An octahedron made of three regular pentagons and five equilateral triangles. I’ve never seen this shape before and don’t know if it has a name. It will make a nice addition to the Periodic Table of Polyhedra I’m compiling. The goal of that project is to identify every topologically unique combination of edges and vertices for polyhedra (mainly low polyhedra –complete up to Octahedra, as coincidence would have it, and selected interesting ones above that. Variations in angles and edge lengths don’t matter for the purpose of this enumeration.)
So I went ahead and made it out of origami. It has a pretty neat folding sequence and symmetry in the layout. I don’t use Robert Lang’s Reference Finder but instead rely on ratios incumbent in the design to to develop the layout, usually going for maximizing the size of the figure in the paper and still having enough left to make the lock. This one turned out very good in the usage department, although I may have to tweak the lock itself a bit.
This shape has the interesting property that if you replace each pentagon with five equilateral triangles you get an icosahedron. Several other shapes have this property, including pentagonal antiprism and a pentagonal pyramid, so they constitute a set. There’s another with 15 triangles and one pentagon, and yet another still with two pentagons and 10 triangles, but it’s not an antiprism because the pentagons are not parallel.
I’m thinking it’d be fun to do this whole series, especially if I can get the unit edge to be the same size across all the different models. Meanwhile, here is the CP and some pics of the a first (not too neat) version of the model.